（ラッセルのパラドクスよりも）もっと古いパラドクス（論理的矛盾）があり、それらのいくつかは古代ギリシャ人たちに知られており、それらは私の発見したのと同様と私には思われる問題を引き起こした。ただし、私の後に続いた著者たちは、それらを（ラッセルのパラドクスとは）異なった種類のパラドクスであると考えた。それらのパラドクスのなかで最もよく知られているものは、クレタ人のエビメニデスについてのパラドクスであった。（即ち）彼は「全てのクレタ人は嘘つきである」と言い、そうしてそう言った時、彼（自分）は嘘をついているかどうかを、人々に答えさせた（caused to ask）。このパラドクスは、もし人が「私は（今）嘘をついている」と言う時、最も単純な形で知られる（理解される）。もし彼が嘘をついているならば、「彼が嘘をついている」ということは嘘であり（嘘となり）、従って彼は真実（本当のこと）を言っていることになる。しかし、彼が真実を言っているのならば、彼は嘘をついている（ことになる）。なぜなら、それが彼が自分でやっていると言っていることだからである。このようにして、（論理的）矛盾は不可避となる。この（論理的）矛盾は、聖パウロによって言及されているけれども（原注：「テトス書」第１章第12節）（訳注：「クレテ人のうちのある預言者が「クレテ人は、いつもうそつき、たちの悪いけもの、なまけ者の食いしんぼう」と言っているが・・・」と書かれている）、聖パウロはその矛盾の論理的側面には興味を持たず、異教徒たちは邪悪であるということを示していることのみに興味を持っていた。しかしこのような古代の謎は、数学者達からは自分たちの主題（数学）に何の関係もないものとしてしりぞけるこことが可能であった。けれども、彼ら数学者は（も）最大の基数は存在するかどうか、また、最大の序数（＝順序数）は存在するかどうか、という問いははっきりと無視することはできず、これら二つの問いは、彼ら数学者達を（論理的）矛盾に陥らせた（のである）。最大の序数（順序数）に関する（論理的）矛盾は、私が論理的矛盾（ラッセルのパラドクスを発見する前に、既にブラリ・フォルティによって発見されていたが、彼の発見した事例はずっと複雑なものであった（注：単純なことで矛盾に陥ることがより重要）。そうして、私は（ラッセルのパラドクス発見前は）、自分の推理に何かつまらない間違いがあるのだろうと考えて済ませていた。いずれにせよ、ブラリ・フォルティの発見した矛盾は、私の発見した論理的矛盾よりもずっと複雑なものであったので、一見したところ、それほど破壊的なものには思われなかった。しかし、最終的には、それもまた同様に重大なものであることを私も認めなければならなかった。
Chapter 7: Principia Mathematica: Philosophical Aspects, n.5 There were older paradoxes, some of them known to the Greeks, which raised what seemed to me similar problems, though writers subsequent to me considered them to be of a different sort. The best known of these was the one about Epimenides, the Cretan, who said that all Cretans are liars, and caused people to ask whether he was lying when he said so. This paradox is seen in its simplest form if a man says, ‘I am lying’. If he is lying, it is a lie that he is lying, and therefore he is speaking the truth; but if he is speaking the truth, he is lying, for that is what he says he is doing. Contradiction is thus inevitable. This contradiction is mentioned by St Paul, who, however, is not interested in its logical aspects but only in its demonstration that the heathen are wicked. But such ancient puzzles could be dismissed by mathematicians as having nothing to do with their subject, though they could not well ignore the questions whether there is a greatest cardinal or a greatest ordinal, both of which landed them in contradictions. The contradiction about the greatest ordinal was discovered by Burali-Forti before I discovered my contradiction, but the matter in his case was much more complex, and I had therefore allowed myself to suppose that there was some unimportant error in the reasoning. In any case, his contradiction, being much less simple than mine, seemed prima facie less devastating. In the end, however, I had to admit that it was just as serious.
　Source: My Philosophical Development, chap. 7：1959． 　　
　More info.:https://russell-j.com/beginner/BR_MPD_07-050.HTM

　この状況（論理的パラドクスの発見による動揺）に対して、哲学者達や数学者達は、多種多様な方法で反応を示した。数学的論理学（mathematical logic 記号論理学）が嫌いで、それは何も生み出さない（不毛だ）と非難していたポアンカレ（Jules-Henri Poincare、1854-1912：フランスの数学者、科学哲学者）は、狂喜して叫んだ。「それはもはや何も生まないものではない。それは矛盾を生む（のだ）」と。それはそれで結構であったが、しかし、そう言ったところで問題の解決にはまったくならなかった。ゲオルグ・カントール（Georg Ferdinand Ludwig Philipp Cantor, 1845-1918：ドイツの数学者で素朴集合論の確立者）の仕事をみとめなかった他の数学者のなかには（『不思議の国のアリス』に出てくる）三月兎の解決法を採用したものもいた。（即ち、こう言った。）「その話題にはもう飽きた。話を変えよう（じゃないか）」。この解決法もまた私には不十分だと思われた（注：皮肉）。けれども、しばらして、数学的論理学を理解し、かつ、論理学の言葉で（in terms of logic 論理学の観点から）問題を解決することが是非とも必要であると認識した人々によって、まじめな解決の試みがなされることになった。これらの試みの最初のものは、たF・P・ラムゼイ（Frank Plumpton Ramsey, 1903-1930：ケンブリッジ大学出身の数学者）の試みであったが、彼は不幸にも早死にして仕事を未完成のままに残した。しかし『プリンキピア・マテマティカ（数学原理）』の出版に先だつ数年間、私はそうした人々の後の（＝『プリンキピア・マテマティカ』出版後の）解決の試みを知る便宜をもたず、事実上、文字通り、困惑の中にただひとり捨ておかれたのである。（注：そういった解決法はもちろん『プリンキピア・マテマティカ』出版後に出てきたもの。みすず書房版の野田氏の訳し方だと早とちりの人は誤解しそう。）

Chapter 7: Principia Mathematica: Philosophical Aspects, n.4 At first I thought there must be some trivial error in my reasoning. I inspected each step under a logical microscope, but I could not discover anything wrong. I wrote to Frege about it, who replied that arithmetic was tottering and that he saw that his Law V was false. Frege was so disturbed by this contradiction that he gave up the attempt to deduce arithmetic from logic, to which, until then, his life had been mainly devoted. Like the Pythagoreans when confronted with incommensurables, he took refuge in geometry and apparently considered that his life’s work up to that moment had been misguided. For my part, I felt that the trouble lay in logic rather than in mathematics and that it was logic which would have to be reformed. I was confirmed in this view by discovering a recipe by means of which a strictly infinite number of contradictions could be manufactured. Philosophers and mathematicians reacted in various different ways to this situation. Poincare, who disliked mathematical logic and had accused it of being sterile, exclaimed with glee, ‘it is no longer sterile, it begets contradiction’. This was all very well, but it did nothing towards the solution of the problem. Some other mathematicians, who disapproved of Georg Cantor, adopted the March Hare’s solution: ‘I’m tired of this. Let’s change the subject.’ This, also, appeared to me inadequate. After a time, however, there came to be serious attempts at solution by men who understood mathematical logic and realized the imperative necessity of a solution in terms of logic. The first of these was F. P. Ramsey, whose early death unfortunately left his work incomplete. But during the years before the publication of Principia Mathematica, I did not have the advantage of these later attempts at solution, and was left virtually alone with my bewilderment.
　Source: My Philosophical Development, chap. 7：1959． 　
　More info.:https://russell-j.com/beginner/BR_MPD_07-040.HTM

　当初私は、自分の推理に何かつまらない間違いがあるにちがいないと考えた。私は推理の各手順（ひとつひとつのステップ）を、論理的顕微鏡（under a logical microscope）で詳しく点検した。しかし、間違いを何も発見できなかった。私はこのことについて、フレーゲ（Friedrich Ludwig Gottlob Frege, 1848- 1925：ドイツの論理学者、数学者で、数理論理学の祖）に手紙を書いた。彼の返事には、算術がよろめき始めており、また、自分の(『算術の基本法則』の)第五法則は間違っていることがわかった、と書かれていた。フレーゲはこの矛盾（ラッセルのパラドクス）によって、非常に落胆させられ、算術を論理学から演繹する企て －それまで自分の生涯の主な目標としていたもの－ を断念した。通約不可能性（注：incommensurables 共約不可能性。体系、概念、方法論などに違いを持つ異なる体系（パラダイム）同士の間で、概念間の対応付けがうまく出来ない状態のこと）（という難物）に直面したピタゴラス学派の人々のように、フレーゲは幾何学の中へ逃避し、どうやら（apparently）それまでの自分の生涯の仕事は見当違いであったと考えたらしい。私はどうかといえば、困難の原因（trouble）は数学の中によりもむしろ論理学の中にあり、改めらるぺきは論理学の方であると感じた。私は、厳密に無限数の矛盾をつくり出すことのできる方法の発見によって、この見解（の正しさ）を確信した。

Chapter 7: Principia Mathematica: Philosophical Aspects, n.4
At first I thought there must be some trivial error in my reasoning. I inspected each step under a logical microscope, but I could not discover anything wrong. I wrote to Frege about it, who replied that arithmetic was tottering and that he saw that his Law V was false. Frege was so disturbed by this contradiction that he gave up the attempt to deduce arithmetic from logic, to which, until then, his life had been mainly devoted. Like the Pythagoreans when confronted with incommensurables, he took refuge in geometry and apparently considered that his life’s work up to that moment had been misguided. For my part, I felt that the trouble lay in logic rather than in mathematics and that it was logic which would have to be reformed. I was confirmed in this view by discovering a recipe by means of which a strictly infinite number of contradictions could be manufactured.
　Source: My Philosophical Development, chap. 7：1959． 　
　More info.:https://russell-j.com/beginner/BR_MPD_07-040.HTM

（発展の方向の）不愉快な側面は、疑いもなくとても不愉快なものであった。（即ち、）学派の区別なく,全ての論理学者が、アリストテレスの時代以来ずっと受け入れてきた前提から、（いろいろな）矛盾が演繹され（導出され）、何かがまちがっていることを示しているがしかしどそれらの問題をどうやって正したらよいかについては何も示していないように思われた。私が享受しつつあった論理的蜜月（logical honeymoon）を終わらせたのは、1901年の春に、そういう矛盾のびとつを私が発見したことであった（注：これが論理学史上有名な「ラッセルのパラドクス」の発見）。私はこの不幸をホワイトヘッドに伝えた彼は「喜びに満ち自信が溢れる朝は再び来らず（never glad confident morning again 喜ばしい確固とした朝は二度と来ないのだ！）」という句を引いて私をなぐさめようとしたが、無駄であった。 　私がこの矛盾に導かれたのは、最大の基数（cardinal number）は存在しないというカントールの証明を吟味することによってであった。私は、無邪気にも、世界にある全てのものの数（総数）は最大の数であるに違いないと信じ、カントールの証明をこの数に適用し、どういう結果が出てくるかを見ようとした。このプロセス（手順/処置）は、非常に奇妙な集合の考察へと私を導いた。それまで正しいと思っていたやり方で考えを進めてゆくと、一つの集合が、ある時は自己自身（その集合自身）の要素（成員/メンバー）でありかつ（同時に）自己自身の要素でないようにと思われた。たとえばティースプーン（紅茶やコーヒー用のスプーン）の集合は、ティースプーン（自体）ではない。しかしティースプーンでないものの集合は、ティースプーンでない集合(one)のひとつである。さらにこのように否定的なものではないところの例も存在すると思われた。たとえば、全ての集合の集合は、ひとつの集合である。ところでカントールの議論（論証）を適用していって、私は、「自己自身の要素でない諸集合」を考えるに至ったが、これらの集合もまたひとつの集合を形づくらなければならないと思われた。そこで私は、この集合がそれ自身の要素であるかないか、自問した。もしそれがそれ自身の要素であるならば、それは、その集合の定義をなしている特性、すなわち、それ自身の要素でないという特性をもたらざるを得ない。逆にもしそれがそれ自身の要素でないとするなら、それはその集合の定義をつくっている特性をもってはならないのだから、それは、それ自身の要素でなければならない。このようにして、二つの可能性のいずれをとっても、それは自身の反対に導き、従って矛盾に陥ることになる。

Chapter 7: Principia Mathematica: Philosophical Aspects, n.3 The unpleasant aspect was indubitably very unpleasant. It appeared that, from premisses which all logicians of no matter what school had accepted ever since the time of Aristotle, contradictions could be deduced, showing that something was amiss but giving no indication as to how matters were to be put right. It was the discovery of one such contradiction, in the spring of 1901 , that put an end to the logical honeymoon that I had been enjoying. I communicated the misfortune to Whitehead, who failed to console me by quoting, ‘never glad confident morning again’. I was led to this contradiction by considering Cantor’s proof that there is no greatest cardinal number. I thought, in my innocence, that the number of all the things there are in the world must be the greatest possible number, and I applied his proof to this number to see what would happen. This process led me to the consideration of a very peculiar class. Thinking along the lines which had hitherto seemed adequate, it seemed to me that a class sometimes is, and sometimes is not, a member of itself. The class of teaspoons, for example, is not another teaspoon, but the class of things that are not teaspoons, is one of the things that are not teaspoons. There seemed to be instances which are not negative: for example, the class of all classes is a class. The application of Cantor’s argument led me to consider the classes that are not members of themselves; and these, it seemed, must form a class. I asked myself whether this class is a member of itself or not. If it is a member of itself, it must possess the defining property of the class, which is to be not a member of itself. If it is not a member of itself, it must not possess the defining property of the class, and therefore must be a member of itself. Thus each alternative leads to its opposite and there is a contradiction.
　Source: My Philosophical Development, chap. 7：1959． 　
　More info.:https://russell-j.com/beginner/BR_MPD_07-030.HTM

『プリンキピア・マテマティカ(数学原理)』の第一の目標（目的）は、純粋数学の全体が純粋に論理学的な前提から帰結し（導出し）かつ、（それは）論理的名辞（logical terms 論理項）によって定義される概念のみを用いる、ということを示すことにあった。これは、もちろん、カントの学説に反対の主張であった。ゲオルグ・カントールは、カントを「あの理屈をこねるフェリシテ人（門外漢／俗物／教養のない者）」と呼び、さらに意味をはっきりさせるために「数学をほとんど知らないところの」とつけ加えたが、私は当初この研究（『プリンキピア・マテマティカ』の執筆）を、カントに対する反駁につけられる一つの付加的説明(as a parenthesis)だと考えていた。しかし時が経つにつれ、この研究（仕事）は二つの異なる方向に発展していった。（即ち）数学的側面においては、全く新たな主題(whole new subjects)が明るみになり（came to light）、新たなアルゴリズム（数学的な問題を解くための一連の手順）を必要とし、それはそれまで冗長で不正確な日常言語にゆだねられていた諸問題を、記号的にとり扱うことを可能にした（のである）。哲学的側面においては、二つの反対（方向）－ひとつは嬉しい、もうひとつは嬉しくない－ 発展があった。嬉しい方向というのは、その仕事（研究）に必要とする論理的装置が、私が以前予想していたよりも小さいものだと判明したことであった。とりわけ、集合（classes）という概念が不要であることがわかったことであった。（私の前著の）『数学の諸原理』においては、一（個）として見られた集合と、多（数）として見られた集合との区別について、多くの議論が存在していた。けれどもこの議論の全体は、この本(前著)に含まれた多くの複雑な（こみ入った）議論と共に、不要であることがわかった(のである)。その結果、『プリンキピア・マテマティカ（数学原理）』は、その最終形においては、不明瞭ということを最も容易に目立つ特徴とするところの哲学的深遠さなるものを欠いているように一見みえたのである（注：非常に明晰なものとなった、ということ／「曖昧に書けば深遠に見える」と感じる人への皮肉）。

Chapter 7: Principia Mathematica: Philosophical Aspects, n.2 The primary aim of Principia Mathematica was to show that all pure mathematics follows from purely logical premisses and uses only concepts definable in logical terms. This was, of course, an antithesis to the doctrines of Kant, and initially I thought of the work as a parenthesis in the refutation of ‘yonder sophistical Philistine’, as Georg Cantor described him, adding for the sake of further definiteness, ‘who knew so little mathematics’. But as time went on, the work developed in two different directions. On the mathematical side, whole new subjects came to light, involving new algorithms making possible the symbolic treatment of matters previously left to the diffuseness and inaccuracy of ordinary language. On the philosophical side, there were two opposite developments, one pleasant and the other unpleasant. The pleasant one was that the logical apparatus required turned out to be smaller than I had supposed. More especially, classes turned out to be unnecessary. In The Principles of Mathematics there is a lot of discussion about the distinction between a class as one and a class as many. The whole of this discussion, along with a great many complicated arguments contained in that book, proved unnecessary. The consequence was that the work in its final form appeared to lack that philosophic profundity of which obscurity is the most easily recognizable feature.
　Source: My Philosophical Development, chap. 7：1959． 　
　More info.:https://russell-j.com/beginner/BR_MPD_07-020.HTM

　1900年から1910年までの間ずっと、ホワイトヘッドと私の二人は、我々の時間のほとんど（bulk of 大部分）を、最終的に『プリンキピア・マテマティカ（数学原理）』(Principia Mathematica)となったもの（仕事）に費やした。この著作の第３巻は、1913年まで出版されなかったが（1913年にようやく出版されたが）、この本を書く仕事は － 校正刷読みは別として－ 1910年に全ての原稿を私達がケンブリッジ大学出版部にもって行った時に終っていた。(これより先)1902年5月23日に書きあげた『数学の諸原理』(The Principles of Mathematics)は、後の著作（『プリンキピア・マテマティカ』の粗雑かなり未熟な下書きであることがわかったけれども、それは後の本（『プリンキピア・マテマティカ』）とはちがって、他の（自分とは立場や考え方が異なる）数理哲学（数学の哲学）との論争を含んでいた。 　我々が論争しなければならない問題は二種類、即ち、哲学的問題と数学的問題の２つがあった。大雑把に言うと、ホワイトヘッドは哲学的問題を私に任せた。数学的問題については、ホワイトヘッドがその記法（notation 記号法）の大部分を、ペアノ（が考案したもの）から借りたものは別として、考案した。（数学的問題については）私は系列(series)に関する仕事の大部分をやり、ホワイトヘッドはそれ以外の大部分をやった。しかしこれは初稿についてのみあてはまる（applies to そうである／該当する）。各部とも、三度書き直された。我々二人のどちらかが初稿を書きあげる、それを相手に送ると、受け取った方はかなり修正を行う（行った）。それが終ると、初稿を書いた方が、決定版（最終稿）に書き直した。三巻全体にわたって、二人の協同の成果（産物）でないものはほとんど一行も存在していない。
Chapter 7: Principia Mathematica: Philosophical Aspects, n.1 Throughout the years from 1900 to 1910 both Whitehead and I gave the bulk of our time to what ultimately became Principia Mathematica, Although the third volume of this work was not published until 1913, our part in it (apart from proof-reading) was finished in 1910 when we took the whole manuscript to the Cambridge University Press. The Principles of Mathematics which I finished on May 23, 1902, turned out to be a crude and rather immature draft of the subsequent work, from which, however, it differed in containing controversy with other philosophies of mathematics. The problems with which we had to contend were of two sorts: philosophical and mathematical. Broadly speaking, Whitehead left the philosophical problems to me. As for the mathematical problems, Whitehead invented most of the notation, except in so far as it was taken over from Peano; I did most of the work concerned with series and Whitehead did most of the rest. But this only applies to first drafts. Every part was done three times over. When one of us had produced a first draft, he would send it to the other, who would usually modify it considerably .After which, the one who had made the first draft would put it into final form. There is hardly a line in all the three volumes which is not a joint product.
　Source: My Philosophical Development, chap. 7：1959． 　
　More info.:https://russell-j.com/beginner/BR_MPD_07-010.HTM

　私が既に言及した事柄に加えて、ペアノとその弟子たちの仕事のなかで（in the work 仕事において）私を喜ばせた点は他にもいろいろあった。私は彼らが図形(figures)を用いないで幾何学を展開し、そうして（thus 結果として/従って）カントの（言う）直観(Anschauung)が必要ないことを明示しているやり方を好んだ。また私は（一つの平面積の）全領域を満たすペアノの曲線(curve)を好んだ（注：ウィキペディアに「ペアノ曲線」に関する説明がありますので、興味のある方はお読みください）。しかし、ペアノに出会う前は、私は関係（というもの）の重要性を痛感していたので、ペアノに会うとすぐに(all at once)、関係の論理を記号的に扱うことによって、彼（ペアノ）の仕事を補う作業にとりかかった。私がベアノに会ったのは(1900年)７月末であり、私が彼が編集している雑誌に発表した関係の論理についての論文を執筆したのは９月であった。私は同じ年の10月、11月、12月を『数学の諸原理』（The Principles of Mathematics）に執筆に費やした。（出版された）この本の第３，４、５，６の各章は、この３ケ月の間に私が書いたほとんどそのままのものである。けれども、第１，２，７章は後に書き直した。私は『数学の諸原理』の初稿を19世紀の最後の日、つまり1900年12月31日に書きあげた。その年の７月以降の月々は、私がそれ以前にもそれ以後にも決して経験したことのないような知的蜜月（intellectual honeymoon）であった。私は毎日、前日まで理解していなかったことを理解している自分を発見した。私は（数学の原理に関する）あらゆる困難が取り除かれ、あらゆる問題が片づいたと思った。しかし、知的蜜月は続かず、翌年（1901年）の始めには、知的な悲しみが私にたっぷり（in full measure）ふりかかってきた。
Chapter 6: Logical Technique in Mathematics, n.10
In addition to the matters that I have already mentioned, there were other things that delighted me in the work of Peano and his disciples. I liked the way in which they developed geometry without the use of figures, thus displaying the needlessness of Kant’s Anschauung and I liked Peano’s curve that filled a whole area. But before coming across Peano I had been filled with the importance of relations, and I therefore set to work almost at once supplementing what he had done by a symbolic treatment of the logic of relations. It was at the end of July that I met him, and it was in September that I wrote a paper on the logic of relations which was published in his journal. I spent October, November and December of that same year on The Principles of Mathematics, Parts III, IV, V and VI of that book are almost exactly as I wrote them during those months. Parts I, II and VII, however, I re-wrote later. I finished this first draft of The Principles of Mathematics on the last day of the nineteenth century – i.e. December 31, 1900. The months since the previous July had been an intellectual honeymoon such as I have never experienced before or since. Every day I found myself understanding something that I had not understood on the previous day. I thought all difficulties were solved and all problems were at an end. But the honeymoon could not last, and early in the following year intellectual sorrow descended upon me in full measure. 　
　Source: My Philosophical Development, chap. 6：1959． 　
　More info.:https://russell-j.com/beginner/BR_MPD_06-100.HTM

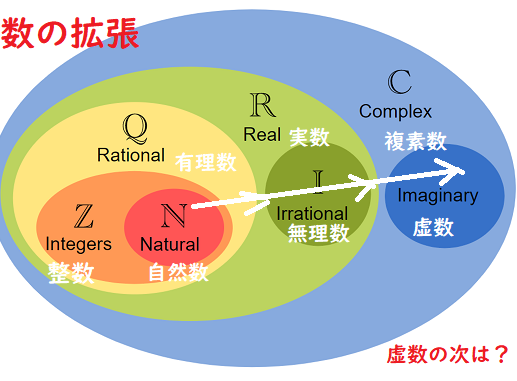
　上記のように整数（whole numbers）を定義すれは、数学が必要とする(数の)拡張（注：整数→分数→有理数→無理数→実数→虚数→複素数といった拡張のこと）には何の困難も存在しない（まったく困難はない）。(即ち)有理分数（rational fractions 分母・分子ともが有理数あるいは多項式である分数）とは、整数の間の関係で,乗法（掛け算）に由来するものである。（注：分数 n/m は割り算 n ÷ m の商、あるいは単位分数 1/mのn倍の数と捉えることができる。／みすず書房版の訳書で、野田又夫氏は「rational fractions」を「分数」と訳しているが、「二つの整数の間の関係」と書いているのであるから、分母あるいは分子に無理数がくる分数は除かなければならない。）実数とは、0からある点までの間に存在する全ての有理数の集合である。たとえば、2の平方根とは、その平方が2よりも小さい全ての有理数である。この定義をはじめて発見したのは私であったと思うが、この定義はピタゴラスの時代以来、数学者を悩まし続けてきた難問を片づけてしまう。複素数（注：実数の単位1と，i2(二乗) ＝－1 であるような虚数単位 i との2つの単位によって構成される数。任意の複素数 z は，a，b を実数として z ＝ a ＋ bi の形に書かれる）は、実数の対（つい）とみなすことができ、このとき、対という語の意味の中には、第一項と第二項があるという意味、即ち,それ（その対）においては項の順序が絶対不可欠であるという意味、が含まれている。
Chapter 6: Logical Technique in Mathematics, n.9
Having defined the whole numbers as above, there is no difficulty in the extensions that mathematics requires. Rational fractions are relations between whole numbers which are derived from multiplication. Real numbers are sets of rational numbers consisting of everything above zero until a certain point. For example, the square-root of two is all rational numbers whose square is less than two. This definition, of which I believe I was the inventor, puts an end to a puzzle which had perplexed mathematicians ever since the time of Pythagoras. Complex numbers can be regarded as couples of real numbers, using ‘couple’ in the sense in which there is a first term and a second term – i.e. in which the order of the terms is essential.
　Source: My Philosophical Development, chap. 6：1959． 　
　More info.:https://russell-j.com/beginner/BR_MPD_06-090.HTM

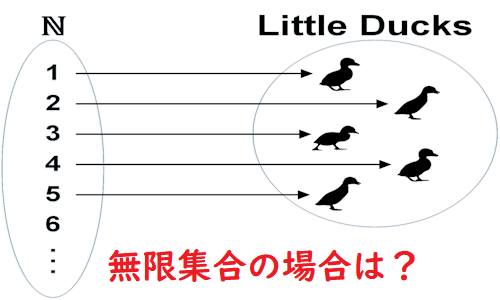
上記の数の定義（法）は、きわめて重要な、さらなるもうひとつの利点を提供する。その利点というのは、無限数に関する多くの困難（問題）に終止符を打つということ（利点）である。項（terms）を一つずつ取りあげて数えることから数が得られると考えられていた間は(While numbers were derived from counting …)、一度に一つずつとって（one at a time）枚挙しつくことのできないような集合の（要素である）数を思い描くことは困難であった。たとえば，我々は、数えることによって、有限数（finite numbers）の終り（最後の数）に到達することは不可能である。どれほど数え続けても、常にそれより大きな数が存在している。それゆえ、数は数えることから生ずると考えられていた間は、有限数が（最大）いくつあるかについて語ることは不可能であると思われた。けれども、今や、数えることはある一つの集合の中にどれだけ多くの項があるかを見出すための単なる一つの方法にすぎず、それはたまたま（数が）有限であるような集合にのみ適用しうるものであるこように思われる。数えることのロジック（論理）を、新しい学説に適合するように考え直すと、次のようになる。たとえば、いまポンド紙幣（pound notes）を数えているとしてみよう。意志行為によって（act of will 無意識にではなく意志をもって）、我々は数枚の紙幣と、数 l、2、3等々との間に、一対一の関係をつけ、もう残った紙幣がないところまで対応付けを行う。そうすると(then)上記の我々の定義（法）に従い（in accordance with）、紙幣の数は、あなたが言及してきた数の数（number of numbers）と同一であることがわかる（注：1,2,3,4,5と5まで数えてもう紙幣が残っていなければ「５」がその数になるということ）。そして、もし我々は１から数え始めて、どの数をもスキップしないで進んだのであれば、言及した数の数は、最後に挙げた数である（注：数え方はいろいろあるためにこのように言っている）。このやり方を、無限集合に適用することはできない。なぜなら、我々の生命はそれほど長くないからである。しかし数えるということはもはや絶対に必須ではないので、そのことはまったく気にする必要はない。（that need cause you no concern）

Chapter 6: Logical Technique in Mathematics, n.8
The above definition of numbers offers a further advantage which is of profound importance, and that is that it puts an end to difficulties concerning infinite numbers. While numbers were derived from counting, which takes terms one by one, it was difficult to conceive of the numbers of collections which could not be exhaustively enumerated one at a time. You cannot, for example, come to an end of the finite numbers by counting: however long you go on, there are always larger numbers to come. Therefore, so long as numbers were derived from counting, it seemed impossible to speak of the number of finite numbers. Now, however, it appears that counting is only one way of discovering how many terms there are in a collection, and is only applicable to such collections as happen to be finite. The logic of counting, as fitted into the new theory, is as follows: suppose, for example, that you are counting pound notes. By an act of will, you establish a one-one relation between the several notes and the numbers 1, 2, 3, etc., until there are no notes left. You then know, in accordance with our definition, that the number of notes is the same as the number of numbers that you have mentioned, and, if you have begun with 1 and gone on without skipping, the number of numbers that you have mentioned is the last number that you have mentioned. You cannot apply this process to infinite collections because life is not long enough. But, as counting is no longer essential, that need cause you no concern.
　Source: My Philosophical Development, chap. 6：1959． 　　　　
　More info.:https://russell-j.com/beginner/BR_MPD_06-080.HTM

　この定義(の仕方)は、種々の利点(advantages)がある。それは、以前、0と1とについて生じていた問題全てを片づけてしまう（deals with 処理する）。(即ち，この定義によれば) 0とは、要素をもたない集合の集合である。つまり、それは（0とは）その唯一の要素が、要素をまったく持たない（一つの）集合であるところの集合である。1とは、ある項 x と同一である任意の項からなるという特性（属性）を持つ集合の集合である。(この定義法の)第二の利点は、一と多に関する困難を乗り越える（gets over）という点である。数えられる項は、一つの命題関数の例（instances 事例）として数えられるので、それに伴う（含まれる）統一性(the unity involved)は、もっぱら「命題関数（として）統一性であり、それは決して例(事例)の多数性とは衝突（矛盾）しない。しかし、これら二つの利点のいずれよりも重要なのは、我々が形而上学的存在者としての数を排除できるということである。数は，実際、単なる言語上の便利なもの（便宜的なもの）にすぎないものとなり、「その他/等々」（etc）とか つまり/即ち（i.e）とかいう略字のもつ以上の実体性を持つものではなくなる。クロネッカー(注：1823-1891、ドイツの数学者)は、数学について哲学的思索を行い、「神が整数をつくり、数学者がその他の数学的道具立てをつくった」と言った。彼が言おうとしたのは、全ての整数は独立の存在者でなければならないが、他の種類の数は独立した存在である必要（性）はない、ということであった。(しかし)上に述べた数の定義（法）により、整数の持つこのような特権は消滅し、数学者の基本的な道具立て（primitive 基本の，根本の）は、「あるいは（or）」とか「ない（not)」とか「全ての（all）」とか「ある（some）」とかいうような純粋に論理的な名辞（論理項）に還元されるのである（減少させられる）。これが、一定の知識の体系において、未定義の術語（terms）及び証明されていない命題の数を減らすために、オッカムの剃刀がいかに有用であるか、ということについての私の最初の経験であった。
Chapter 6: Logical Technique in Mathematics, n.7
This definition has various advantages. It deals with all the problems that had previously arisen concerning 0 and 1. 0 is the class of those classes that have no members – i.e. it is the class whose only member is a class having no members. 1 is the class of those classes that have the property of consisting of whatever is identical with some term x, A second advantage of the definition is that it gets over difficulties concerning the one and the many. Since the terms counted are counted as instances of a propositional function, the unity involved is only that of the propositional function, which in no way conflicts with the plurality of instances. But much more important than either of these two advantages is the fact that we get rid of numbers as metaphysical entities. They become, in fact, merely linguistic conveniences with no more substantiality than belongs to ‘etc.’ or ‘i.e.’. Kronecker, in philosophizing about mathematics, said that ‘God made the integers and the mathematicians made the rest of the mathematical apparatus’. By this he meant that each integer had to have an independent being, but other kinds of numbers need not have. With the above definition of numbers this prerogative of the integers disappears and the primitive apparatus of the mathematician is reduced to such purely logical terms as or, not, all and some. This was my first experience of the usefulness of Occam’s razor in diminishing the number of undefined terms and unproved propositions required in a given body of knowledge.
　Source: My Philosophical Development, chap. 6：1959． 　
　More info.:https://russell-j.com/beginner/BR_MPD_06-070.HTM