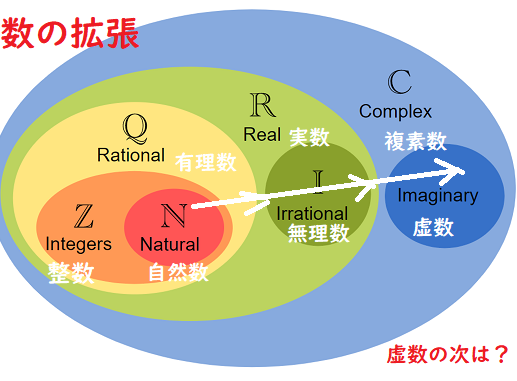
上記のように整数（whole numbers）を定義すれは、数学が必要とする(数の)拡張（注：整数→分数→有理数→無理数→実数→虚数→複素数といった拡張のこと）には何の困難も存在しない（まったく困難はない）。(即ち)有理分数（rational fractions 分母・分子ともが有理数あるいは多項式である分数）とは、整数の間の関係で,乗法（掛け算）に由来するものである。（注：分数 n/m は割り算 n ÷ m の商、あるいは単位分数 1/mのn倍の数と捉えることができる。／みすず書房版の訳書で、野田又夫氏は「rational fractions」を「分数」と訳しているが、「二つの整数の間の関係」と書いているのであるから、分母あるいは分子に無理数がくる分数は除かなければならない。）実数とは、0からある点までの間に存在する全ての有理数の集合である。たとえば、2の平方根とは、その平方が2よりも小さい全ての有理数である。この定義をはじめて発見したのは私であったと思うが、この定義はピタゴラスの時代以来、数学者を悩まし続けてきた難問を片づけてしまう。複素数（注：実数の単位1と，i2(二乗) ＝－1 であるような虚数単位 i との2つの単位によって構成される数。任意の複素数 z は，a，b を実数として z ＝ a ＋ bi の形に書かれる）は、実数の対（つい）とみなすことができ、このとき、対という語の意味の中には、第一項と第二項があるという意味、即ち,それ（その対）においては項の順序が絶対不可欠であるという意味、が含まれている。
Chapter 6: Logical Technique in Mathematics, n.9
Having defined the whole numbers as above, there is no difficulty in the extensions that mathematics requires. Rational fractions are relations between whole numbers which are derived from multiplication. Real numbers are sets of rational numbers consisting of everything above zero until a certain point. For example, the square-root of two is all rational numbers whose square is less than two. This definition, of which I believe I was the inventor, puts an end to a puzzle which had perplexed mathematicians ever since the time of Pythagoras. Complex numbers can be regarded as couples of real numbers, using ‘couple’ in the sense in which there is a first term and a second term – i.e. in which the order of the terms is essential.
　Source: My Philosophical Development, chap. 6：1959． 　
　More info.:https://russell-j.com/beginner/BR_MPD_06-090.HTM