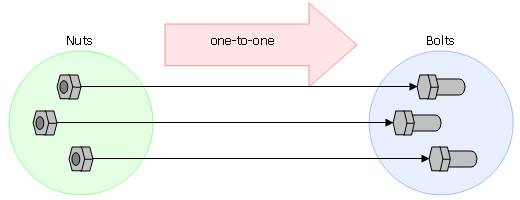
数についての(ペアノ)以前の理論は、0と1とについて、常に困難に陥った(陥っていた)。そして、まず私が感心したのは、これらの困難を扱うペアノの手腕であった。しかし、この新しい観点の全帰結を私が引き出したのはそれからかなり経ってからのことであった。数学において(は)「集合」(classs)について考えることは便利であり(であるので)、長い間私は集合と命題関数とを区別する必要があると考えていた。けれども、最終的にはこの区別が技術的工夫として以外には不必要であるとの結論に達した。「命題関数」という句は、多分、不必要に恐ろしげに(formidable)聞こえるかも知れない。多くの場合、それは「特性(属性)」(property)という語で言いかえることが可能である。その結果として(thus それゆえ)我々は、各々の数は諸特性のなかの一特性(a property of certain properties)であると言ってよいであろうが、しかし、最終的な分析における場合以外は、「集合」(class)という語を使い続けることが、いっそう容易であろう。 上記の考察によって私が導かれた数の定義は、それよりも16年も早く(前に)、フレーゲによって定式化されていた(はっきりと述べられていたが、私はそのことを、私がそれを再発見したおよそ一年後まで知らなかった。私は(数の)2を「あらゆる対(couple つい)の集合」と定義し、(数の)3を「あらゆる三つ組(trio)の集合」等々、と定義した。「対」というのは、xとyという要素をもつ集合であって、xとyとは同一でなく、かつもし第三者zがその集合の要素であるなら、zはxかyのいずれかと同一であるような集合である。一般に一つの数とは、「類似性(相似性)」(similarity)と言われる特性(属性)をもつところの,集合の集合(a set of classes)である。「類似性(相似性)」は次のように定義される。即ち、二つの集合はそれらの諸項(terms)を一つずつ対(つい)にする方法がある(存在する)とき、類似的(相似)であると言われる。たとえば、一夫一婦制の国においては(in a monogamous country)、結婚している男の数は結婚している女の数と同数であるということを、男女がそれぞれ何人いるかを知らずとも、知ることが可能である(注:現代では結婚が男女の組み合わせ以外もあるようになったため、このように断言できなくなっている)。(私は夫を失った妻や妻を失った夫を勘定に入れていない。) さらにまた、一人の人が片足を失っていないなら、彼のもっている右足の靴の数は、左足の靴の数と同じであることはかなりの場合確かであろう。また、ある会の出席者が皆椅子に坐っており、かつ空いた椅子が一つもなければ、椅子の数は椅子に坐っている人間の数と同じに違いない。これらすべての場合において、一方の集合の要素と他方の集合の要素との間に、いわゆる一対一の関係が存在するのであり、そういう一対一の関係の存在こそ「類似性」といわれるものである。そこで任意の集合の数とは、その集合に類似な集合の全てであると定義される。
Chapter 6: Logical Technique in Mathematics, n.6 The older theories of number always got into difficulties over 0 and 1, and it was Peano’s capacity of dealing with these difficulties that first impressed me. But it was a good many years before I drew the full consequences of the new point of view. It is convenient in mathematics to think of ‘classes’, and for a long time I thought it necessary to distinguish between classes and propositional functions. Ultimately, however, I came to the conclusion that this distinction is unnecessary except as a technical device. The phrase ‘propositional function’ sounds perhaps unnecessarily formidable. For many purposes one can substitute the word ‘property’. Thus, we may say that each number is a property of certain properties, but, except in ultimate analysis, it is perhaps easier to continue to use the word ‘class’. The definition of numbers to which I was led by the above considerations had been formulated by Frege sixteen years earlier, but I did not know this until a year or so after I had re-discovered it. I defined 2 as the class of all couples, 3 as the class of all trios, etc. A couple is defined as a class of which there are members x and y, x is not identical with y, and, if z is a member of the class, then z is identical with x or with y, A number, in general, is a set of classes having the property which is called ‘similarity’. This is defined as follows; Two classes are similar if there is a way of coupling their terms one to one. For example, in a monogamous country, you can know that the number of married men is the same as the number of married women, without having to know how many there are of either (I am excluding widows and widowers). Again, if a man has not lost a leg, you may be pretty sure that the number of his right-hand shoes is the same as the number of his left-hand shoes. If every member of a company has a chair to sit on and there are no empty chairs, the number of chairs must be the same as the number of people sitting on them. In all these cases there is what is called a one-one relation between the terms of one class and the terms of another, and it is the existence of such a one-one relation which is defined as similarity. The number of any class is defined as all the classes that are similar to it.
Source: My Philosophical Development, chap. 6:1959.
More info.:https://russell-j.com/beginner/BR_MPD_06-060.HTM